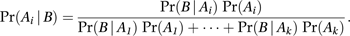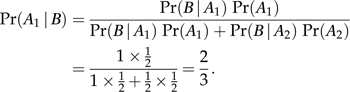Noah's Ark: Test Boat #1

Over the past week or so, I have been attempting to find a way to determine the total volume of every species on the planet that would not be able to swim for the duration of the flood. This has been quite a dubious task, to say the least.

To my dismay, most statistics on animals report only their average mass and height, as opposed to volume (height x length x width). In addition, the male and female species are almost always different sizes, which adds more variables to take into account.

In this post, I will show you my first (and unfortunately very inaccurate) attempt at determining whether or not all of the animals in the present day could have fit on Noah's ark. If anyone has ideas concerning an effective way to determine the total volume of all animals which could not have survived the flood, please let me know.
-----------------------------------------------------
Calculation Attempt #1

Purpose: Determine if the number of 'animals' alive today could have fit on the ark (the ark's volume is 41006.25 cubic meters) (see http://honestsearchfortruth.blogspot.com/2011/09/how-big-was-noahs-ark.html for how I got this volume)

Methods: Use low estimations of the average volume needed for each category of life as opposed to calculating the volume needed for each individual species. A low estimation is being used for the following reason: If the low volume would not fit on the ark, then the actual volume (which is higher than this estimation) also would not fit on the ark.

'Animals' will be divided into the following broad categories:

1. Mammals: 4,475-5,000 species
2. Birds: 9,000-10,000 species
3. Reptiles: 7,984 species
4. Amphibians: 5,400
5. Insects: 1-30 million+ species
6. Arachnids: 75,500 species|

I obtained all of these numbers from <http://animals.about.com/od/zoologybasics/a/howmanyspecies.htm>.

It will be estimated that each category will require on average the following volume:

1. Mammals: 2 shoe boxes (about .01 cubic meters)
2. Birds: 1 shoe box (about .005 cubic meters)
3. Reptiles: 1/2 shoe box (about .0025 cubic meters)
4. Amphibians: 1/2 shoe box (about .0025 cubic meters)
5. Insects: 1 match box (about .0000123 cubic meters)
6. Arachnids: 1 match box (about .0000123 cubic meters)

Note: Your typical Air Jordan shoe box has the dimensions: 29.5cm x 18cm x 9.5cm (.295m x .18m x .095 m). A typical matchbox has the dimensions .0254m x .0381m x .012m.
 
I understand that these volume estimates are a gross oversimplification of the situation. However, I attempted to keep my estimates of volume required per species on the low end of the spectrum (I'm sure you can think of plenty of mammals that would take up more than 2 shoe boxes of volume) (then again, there are many insects and arachnids that would require much less than a match box).

Now, to calculate the volume needed by all of these animals.

1. Mammals: 5000 species x .01 cubic meters x 2 = 100 cubic meters
2. Birds: 10,000 species x .005 cubic meters x 2 = 100 cubic meters
   
3. Reptiles: 7,984 species x .0025 cubic meters x 2 = 40 cubic meters
   
4. Amphibians: 5,400 species x .0025 cubic meters x 2 = 27 cubic meters
   
5. Insects: 15,000,000 species x .000123 cubic meters x 2 = 3690 cubic meters
6. Arachnids: 75,500 species| x .000123 cubic meters x 2 = 18.5 cubic meters

Now, taking the sum of numbers 1 through 6 we get: 3975.5 cubic meters
-----------------------------------------------------

Conclusion:
From the calculations I have just made, no conclusions can be drawn. I made far too many estimations and oversimplifications. This post was more of a first practice run to get acquainted with the subject material and get experience so that the second, third, fourth, etc. attempts will be more accurate.

If all of my assumptions were correct (which they were NOT), then all the animals could have fit in the ark easily:

Compare 3975.5 cubic meters with 41006.25 cubic meters. Or a comparison that is easier to understand: 4,000 cubic meters to 40,000 cubic meters. Basically, all of the animals could have fit inside of the ark ten times over.

Remember that I attempted to utilize low estimations for volume, which will obviously cause the final volume to be lower than it actually is.

Keep in mind that the floors of the decks would also take up some volume, as would the food required to feed the animals for the duration of the journey. There were a number of animals that were taken in 7's instead of 2's. The humans also need to be taken into account. In addition, space would have been required for hallways, packaging of foods, prevention of violence between species, waste disposal, tools, and a number of other things would have also taken up a great deal of space.

At this point, the answer to the question "could all the animals that exist today have fit on the ark?" is 'we don't know, but if we had to bet our lives on it right now, we would go with yes, the animals could fit.'

In the next few posts, I will be attempting to improve my calculations to the point that meaningful conclusions can be drawn.

What Was Noah's Definition of Animals?

Before reading this post, check out part 1 of this series! http://honestsearchfortruth.blogspot.com/2011/09/how-big-was-noahs-ark.html

Here is exactly what God told Noah to take on the ark with him:

Genesis 6:19-21
"You must bring into the ark two of every kind of living creature from all flesh, male and female, to keep them alive with you. Of the birds after their kinds, and of the cattle after their kinds, and of every creeping thing of the ground after its kind, two of every kind will come to you so you can keep them alive. And you must take for yourself every kind of food that is eaten, and gather it together. It will be food for you and for them."

and:

Genesis 7:1-3
'The Lord said to Noah, “Come into the ark, you and all your household, for I consider you godly among this generation. You must take with you seven of every kind of clean animal, the male and its mate, two of every kind of unclean animal, the male and its mate, 7:3 and also seven of every kind of bird in the sky, male and female, to preserve their offspring on the face of the earth."'

Here is what I take from the first passage:

• Two of every living creature from all flesh, male and female (would this exclude organisms that are asexual?)
• Of the birds after their kinds
• Of the cattle after their kinds
• Of every creeping thing of the ground after its kind

Here is what I take from the second passage:

• Seven of every clean animal
• Two of every unclean animal
• Seven of every kind of bird in the sky

There may be a discrepancy between these two lists, but that is the topic of another discussion

For the purpose of examining the topic at hand further, I propose the following list of things which God commanded Noah to take on the ark with him:

• Two of every living creature from all flesh
• Birds
• Cattle
• Creeping things of the ground
• Seven of every kind of clean animal

---------------------------------------------
Now, how did Noah interpret God's command? One thing we can know for certain is that Noah was not a taxonomist, biologist, or zoologist like our modern scientists are. The definition of what we consider to be separate species now is different than what Noah would have considered to be separate species over 4000 years ago. Because of this unknown factor, it is extremely difficult to pin down a reasonable list for what Noah would have felt necessary to take on the ark with him.

Fortunately, my line of argumentation is going to bypass the topic in the previous paragraph.

---------------------------------------------
Conclusion:
We do not know how Noah interpreted God's command. In the next post, we will see why the question 'what did God command Noah to take on the ark' can easily be bypassed.

I addressed this topic because most would consider it to be a relevant question, and because I will need to point out that 'we don't know what Noah's interpretation' was in future posts.

The Flood: Planetary Submersion or Local Phenomenon?

The next step in determining the plausibility of Noah’s ark and the flood is to answer the question “is Genesis referring to the entire planet, or just part of the planet?”

There are several Hebrew words in Genesis 6 that are translated ‘the earth.’

'adamah
Definition:
1) ground, land
1a) ground (as general, tilled, yielding sustenance)
1b) piece of ground, a specific plot of land
1c) earth substance (for building or constructing)
1d) ground as earth's visible surface
1e) land, territory, country
1f) whole inhabited earth
1g) city in Naphtali,

Urah and Urab
Definition:
1) land, earth
1a) earth
1a1) whole earth (as opposed to a part)
1a2) earth (as opposed to heaven)
1a3) earth (inhabitants)
1b) land
1b1) country, territory
1b2) district, region
1b3) tribal territory
1b4) piece of ground
1b5) land of Canaan, Israel
1b6) inhabitants of land
1b7) Sheol, land without return, (under) world
1b8) city (-state)
1c) ground, surface of the earth
1c1) ground
1c2) soil
1d) (in phrases)
1d1) people of the land
1d2) space or distance of country (in measurements of distance)
1d3) level or plain country
1d4) land of the living
1d5) end(s) of the earth
1e) (almost wholly late in usage)
1e1) lands, countries
1e1a) often in contrast to Canaan
--------------------------------------------------

At this point it seems that there are two possible interpretations of ‘the earth’ in Genesis 6.

1. The entire planet
2. A part of the planet

--------------------------------------------------
Much debate could go into which is the better interpretation, but I don’t think it matters. The reason for this is that even if ‘a part of the planet’ is a better interpretation, the water had to be at least deep enough to cover Mount Ararat.

And why, you ask, did I just bring up Mount Ararat? Great question! The answer lies in chapter 7 of Genesis:

Genesis 7:19-24 (Net)
“The waters completely inundated the earth so that even all the high mountains under the entire sky were covered. The waters rose more than twenty feet above the mountains. And all living things that moved on the earth died, including the birds, domestic animals, wild animals, all the creatures that swarm over the earth, and all humankind. Everything on dry land that had the breath of life in its nostrils died. So the Lord destroyed every living thing that was on the surface of the ground, including people, animals, creatures that creep along the ground, and birds of the sky. They were wiped off the earth. Only Noah and those who were with him in the ark survived. The waters prevailed over the earth for 150 days.”
-----------------------------------------


So, the water was more than 20 feet higher than the mountains, and this depth was maintained for 150 days. Mount Ararat has an elevation of 5,137 m (It is the 48th highest peak in the world). This means that even if Genesis 6 is referring to a flood in one part of the world, that flood had to rise up to at least 5,137 m (+ 20 feet) above sea level across the entire planet.

Note: It has not escaped my attention that the height of Mt. Ararat could have changed since the time of the flood. The thing is a volcano, after all (and one which has been active since the time of the flood). I am going to overlook this variable for now and return to it as soon as possible (maybe you could find some geo-historical data and send it to me!)

For now I think it is safe to assume that the water of the flood must have been at least 5000 meters above present day sea level. Because fluids fill the shape of their container, anything on the entire planet under 5000 meters would have been submerged.

Here is a map of the earth with an elevation scale included (see citation). If a flood deep enough to cover Mt. Ararat took place, then the vast majority of the planet would have been covered by water. Only the darkest of the red areas would have stuck out over the surface (If you can't see the numbers on the scale, click on the link at the bottom of the picture).




http://www.eoearth.org/files/120601_120700/120668/620px-Earth_relief_map.jpg

 


What is the point of all of this? Even if the Hebrew words in Genesis for ‘earth’ should be interpreted as a ‘local flood of a specific area,’ that ‘local flood’ would have been over 5000 meters deep. Therefore, basically the entire earth would have been covered by water anyway.
-----------------------------------------------------------

Conclusion:

Question- Is it possible to interpret the flood in Genesis as being a local event?

Answer- No, the water had to have been over 5000 meters deep, which would have submerged basically the entire planet underwater no matter how you interpret Genesis 6.

The best intepretation of 'a flood that covered the earth' in Genesis is defined by Genesis 7: 'a flood that was deep enough to cover all of the mountains in the area by at least 20 feet' (which means that the water was at least that deep everywhere else on the planet as well). A safe bet for this depth is about 5000 meters above present day sea level.

---------------------------------------------
Citation

Michael Pidwirny (Lead Author);Galal Hassan Galal Hussein (Topic Editor) "Mountain". In: Encyclopedia of Earth. Eds. Cutler J. Cleveland (Washington, D.C.: Environmental Information Coalition, National Council for Science and the Environment). [First published in the Encyclopedia of Earth July 18, 2010; Last revised Date July 18, 2010; Retrieved September 12, 2011 <http://www.eoearth.org/article/Mountain>
There are several Hebrew words in Genesis 6 that are translated ‘the earth.’

How Big Was Noah's Ark?

Because I need some time to process the information I have gathered on the historical argument, I will be taking a short break from such topics as the resurrection, the historical Jesus, and the gospel accounts. In the mean time, I will be doing a series on Noah's ark!

If Noah's ark was not big enough to fit as many animals as the Bible claims it did, we could have an external discrepancy on our hands. The first step in making this determination is finding out just how big Noah's ark is.

According to Genesis 6:15-17 (ESV)
"This is how you are to make it: the length of the ark 300 cubits,^[a] its breadth 50 cubits, and its height 30 cubits. ¹⁶Make a roof^[b] for the ark, and finish it to a cubit above, and set the door of the ark in its side. Make it with lower, second, and third decks. ¹⁷ For behold, I will bring a flood of waters upon the earth to destroy all flesh in which is the breath of life under heaven. Everything that is on the earth shall die."

• A cubit is about 18 inches, or 45 centimeters.

And now for some math which is much easier than probability calculus:

Length: 300 cubits x 45 centimeters = 13500 centimeters

13500 centimeters x (1 meter/100 centimeters) = 135 meters long

Breadth: 50 cubits x 45 centimeters = 2250 centimeters

2250 centimeters x (1 meter/100 centimeters) = 22.5 meters wide



Height: 30 cubits x 45 centimeters = 1350 centimeters

1350 centimeters x (1 meter/100 centimeters) = 13.5 meters high

------------------------------------------------------------------

Now what we really want is total volume, so:

135 meters x 22.5 meters x 13.5 meters = 41006.25 cubic meters = Approximate Volume of Noah's Ark*

*It is possible that the corners were curved, as opposed to being a box with flat edges. The would cause a slight variation in the actual volume.

------------------------------------------------------------------

Interestingly enough, someone built a full sized replica of the ark. Here are a few photos of Johan Huibers' full size ark taken from http://www.myinterestingfiles.com/2008/10/working-replica-of-noahs-ark.html.

In the following series of posts, we will examine topics such as:

• What does Genesis claim about the number of animals God told Noah to take on the ark?
• Does Genesis claim that the flood was worldwide, or localized to a specific area?
• Could Noah have gathered all of these animals?
• Could the number of animals claimed in Genesis fit on the ark?
• Is there evidence for or against a worldwide flood?

Guest Post: Further Discussion of the Cosmological Argument

Hi there! My name is Brandon, and Josh has graciously allowed me to post some of my thoughts on his blog. Per his request, here’s just a little about myself before we begin: I’m a grad student at Caltech working on my PhD in Mechanical Engineering. Before that, I attended New Mexico Tech for my BS in ME, and before that, I graduated high school with the best homeschool education anyone could ask for. I love discussing and studying anything having to do with mathematics, physics, computer science, philosophy, or theology. (Or politics, but that’s kind of irrelevant on this blog.) I’ve been a Christian all my life, and I am a strong proponent of intellectual Christianity. I’m an “open theist” (kind of like being an Armenian, but more so) and believe strongly in the home church model. I think that about covers it, so let’s get on to the Cosmological Argument.

The Cosmological Argument (CA) has been discussed previously by Josh, and the conclusion was that it is not a good argument to use. I’d like to offer a slightly different perspective of the CA from the version William Craig used and which was discussed on the blog previously. Although the CA is often misused (for instance, by Craig) it is not without its place in the collection of arguments supporting God’s existence.

I’d like to start off with what the Cosmological Argument is not:

-It is not an argument that is likely to convince anyone of the truth of Christianity.
-It is not an argument that can prove the existence of God.

On the other hand, here’s what the Cosmological Argument is:
-It is scientifically valid.
-It is an argument that can strongly support (or at least, allow for) the existence of God.

I’ll list my version of the CA in more technical terms using a numbered premise format:

1. The Second Law of Thermodynamics states that the amount of entropy in the universe is always increasing. In other words, the amount of “useful energy” is always being used up. (Useful energy means energy that may be harnessed to do work.)

2. If the useful energy in the universe is always being used up, then after an infinite amount of time, it will all be gone.

3. If the universe has always existed for an infinite amount of time, all the usable energy would be gone.

4. The usable energy is not gone yet.

5. Therefore, the universe has not always existed: it must have had a starting point at some point in the past.

(This may seem a bit pedantic—after all, many scientists already believe that the universe started with the “big bang.” Hang with me.)

6. At some point in the past (probably when the universe began), usable energy must have been put into the universe.

7. By the laws of physics, it is impossible for usable energy to be spontaneously generated, so the universe must have undergone a non-physical process when the usable energy was put in.

8. If something is non-physical, it is impossible for us to explain it with the laws of physics. (By definition)

9. We do not know what started the universe, and we cannot explain it using our laws of physics.

William Craig’s mistake here is jumping immediately to “Well, God must have created it!” That is not the conclusion that can be drawn from the last premise! That goes far beyond the scope of this argument.

The real power of the CA is as follows. The scientific community often leads the general population to believe that everything has been figured out and explained. While they have a number of theories about what started the universe, they must all be (as we proved above) unscientific. As long as that is the case, it requires just as much faith to provide a natural explanation for the universe as it does a supernatural one.

The CA does not prove the existence of God, but it demonstrates scientifically that the existence of some supernatural being could provide a very good explanation for the creation of the universe.

And that’s my take on the Cosmological Argument! Thoughts?

The Self: The Spiritual Doorway in the Brain Chapter 3

When you talk about yourself do you really know what you mean? Some people say, “That’s just who I am!” but do they actually understand themselves? These are very important questions and oddly answerable for a neuroscientist.

The self is not a large whole but rather, fragments (or memories) of our past experiences. The self is the most deeply ingrained knowledge we have, which is shown in Alzheimer’s patients. Often they will forget where they are and forget autobiographical information but continue to answer at the call of their names.

Dr. Nelson concedes that there is no good definition for the self in terms of what we know now within neuroscience. He wants to draw a very clear line between the self and consciousness. They are related but they are not the same and therefore we cannot use these terms interchangeably. However, we can use “me” or “I” to refer to the self because these are the terms we use referring to our selves. Studies have shown that other animals besides humans have a sense of self. This group includes primates (obviously), elephants and dolphins. The greatest difference between the human sense of self and other intelligent animals’ is the way we express our selves.

Humans have developed a very specific language to communicate with one another about ideas and experiences. Other animals use communication for different things like warnings, finding another’s location, etc. Foolishly humans think other animals understand our language so we talk to them as if they know our intentions. Humans are by far the most arrogant of the animal world. Other animals also use body language way more than humans do because of the specific language we have. We have made body language almost unnecessary. The animal world revolves around survival. Humans are far beyond that in everyday life (usually) and have been for thousands of years. The focus turned from survival to metaphysical subjects (beyond and outside of ourselves). I like to call this the evolution of mind.

Something that can really shake a person’s sense of self is if they suddenly don’t recognize a part of their body as their own. Wouldn’t that be weird? Some people actually develop this problem!

Suddenly (after a stroke in the right parietal lobe of the brain which is dominant for identifying one’s self in the world) their arm is unrecognizable to them, although they accept that it is there. They understand how crazy it sounds that they don’t know this particular limb, but their brains are unable to accept it as a part of themselves. Past memories of this arm seem to be gone but it is actually the retrieval of these memories that has been shut off (due to the parietal lobe stroke).

Dr. Nelson refers to an interesting phenomenon called the phantom limb syndrome. When someone loses a limb sometimes the patient feels as though the limb is still there and functions just as it normally would. Sometimes, the limb is stuck in an uncomfortable position or it causes pain to its owner.

Why would someone think that their limb is there when they can look down and see that it is clearly not there? The answer is: the part of their brain that controlled that limb or offered sensation and perception to its owner is still there. It doesn’t leave with the limb so sometimes activation of nerves close by will cause feeling to the owner. This phenomenon can be very confusing, although it doesn’t last forever. Eventually, the brain will allocate some other function along the same general lines to the part of the brain that previously controlled the limb (because the brain is freaking cool like that!). Dr. Nelson relates a case study in which a few neuroscientists felt as though they should help to relieve the situation for one particular patient.

A group of neuroscientists were collaborating on one patient’s problem (as they often do because multiple minds are better than one) with a phantom arm that was in an uncomfortable position and was causing him much distress. The neuroscientists developed a contraption that showed a rubber arm where his arm would have been. By having him observe the arm being stimulated over and over again with a feather for a few weeks, he was forever relieved of his phantom limb. His brain registered the stimulation of the arm so that he literally (specifically his brain) felt the sensation in his phantom arm. The contraption quickly aided his brain in pruning (neuro term I always liked) back those neuro-connections that had still been there. This shows that the brain is able to rearrange the self rather fluidly.

Some people believe they have sprouted another invisible or “spare” limb. This is due to a stroke in the brainstem. The spare limb in the case study Dr. Nelson refers to was hostile toward its owner and would try to strangle her. Sometimes this patient would sprout another leg. It is not completely understood how the brain makes this happen but these sensations didn’t last forever. They went away as she recovered from her stroke. The question these examples bring up is: “are the revelatory changes in one’s sense of self during spiritual experiences just kindred illusions?” Dr. Nelson’s opinion is clear on the matter because he states, “If the self could be thought of as a figment of our imaginations, could the same be said of spiritual truth?”(Dr. Nelson).

Another important point in our search is that the frontal lobes of our brains house our personalities. Changes to the prefrontal lobes (whether injury or disease) can cause changes in an individual’s personality. The most famous case of this was a railroad man who was shot through the frontal lobes with a metal rod in a work-related accident. He was known, previously, as a kind, generous and generally happy person but after the accident he became rude and tactless.

The brain is so fascinating because two halves (or hemispheres) form its whole and the mirror images communicate through a dense band of nerve fibers (corpus callosum) near the brain’s center. If the fibers are severed (generally this is done to relieve grand mal seizures) the two hemispheres can still communicate to a degree. However, there is a very clear dispute between the two sides. Interestingly, case studies have shown that often either arm of the subject will do the opposite of the one on task. For example, one patient was observed pulling his pants up with one arm and pulling them down with the other. Dr. Nelson suggests perhaps there may be more than one conscious self with a single brain and begs the religious yet scientifically charged question “Might one side of the brain feel itself blessed while the other thought it was damned?” You could say that’s true.

Sometimes severing the corpus callosum would not relieve a patient of seizures (depending on the location and severity) but removing a portion of the brain would. What happens in this situation is a neuropsychologist investigates what part of the brain to remove. This is done by anesthetizing portions of the brain previous to surgery (totally painless due to the lack of pain sensing neurons [yes, they’re different and for more info, just ask ;-)] directly on the brain’s surface). Then the neuropsychologist asks the patient questions to determine what parts are awake. Often, the patient will exhibit drastically different personality traits as well as when the prefrontal cortex of the brain is injured.

Dr. Nelson states, “It (each hemisphere) may also have its own ideas and experiences of spiritual insight or truth, different thoughts and feelings about the holy and the divine.”

Dr. Nelson deems it important to point out that evolution led to the development of two hemispheres within the vertebrate brain (that’s us). Nature would not separate the brain hemispheres if it weren’t meant for each side to do something a little different than the other (or specialize). We all call ourselves “left brain” or “right brain” people due to what we are naturally proficient in doing. The left hemisphere is good at organization and problem solving with the right focuses on tasks and experiences. The right brain is interested in accuracy of an event and the raw data while the left is interested in making connections or a story about the occurrences.

Dr. Nelson makes a surprising statement in my opinion when he asserts, “It is tempting to speculate that as primates develop their interpretive, language-rich left hemisphere, they began interpreting their observations of nature in a supernatural context. It could be that the left brain first brought us our gods; it certainly allowed us to talk about them.”

How does a person think without language? People can be born without hearing, and before today’s advances, would never hear their whole lives. Some of these people are also born blind like Helen Keller. People couldn’t believe that people like her (before she came along) were people at all because they had no way of communicating (until Anne Sullivan came along). How surprised was everyone when she became an author after discovering the English language? “Does language make the self or does language only make the self detectable? Considerable evidence tells us that the right hemisphere can, on its own, be a human self without language.” The answer: The same way you think with language. Language gives us a common understanding of what we think.

Cotard’s syndrome is named after the doctor who uncovered the first well known case study. The result is that the patient thinks they are dead, although they are perfectly fine and completely rational (besides the obvious). They will often think they are decomposing or walking around in the afterlife. It is very difficult to study something that occurs so infrequently so there is little known about the part of the brain that would cause this malfunction. Dr. Nelson says that when it is found it will be the part that affirms our existence. He states, “The power our brain has over our most important assumptions is awesome. We need to keep this firmly in mind as we examine spiritual experiences such as the mystical and near-death.”

Join me for the next section and chapter four, “The Varieties of Near-Death Experience”.

Don't be Afraid to Comment!

I need you to comment to point out errors in my thinking!!!

Don't be afraid! If you have something to say, either post it or send me a facebook message! I promise that I will be nice! However, I also promise that I will be honest. I may shake your foundation, and may point out a current error in your thinking. But is that bad? No! Having an error pointed out and changing is much better than going on living with an unidentified error!

Example: Spaceship takes off with an unknown error. On the way back to earth, it burns up in the atmosphere because of the unknown error. Would have been much better to have fixed the error! Obviously someone would have been called out... Maybe embarrased a little. But who cares!?

I need you to point out my unkown errors, and I will do the same for you!

"And Take the Sword... of Probability Calculus"

Craig uses probability calculus to attempt to refute Ehrman’s claim that historians “cannot say that the explanation which is inherently the most improbable is probable” (whether or not he succeeded is up to you). For a summary of Ehrman's argument against the resurrection, see: http://honestsearchfortruth.blogspot.com/2011/09/can-miracle-ever-be-best-explanation.html

In the debate, Craig had severe time limitations and could not explain the probability calculus he used enough for most people to understand what the heck he was talking about. Out here in the real world, however, we have as much time as we would like! This means that I will be spending my Saturday morning learning about and explaining the relevant topics in probability calculus for you. This is necessary if we want to make a decision as to whether Craig, Ehrman, or neither of them is correct on the historicity resurrection! To know whether or not Jesus rose from the dead, you need to know a little probability calculus. 

I promise that I will only share the math that is absolutely necessary for understanding! Don’t be intimidated by the subject material of this post- it is pretty easy if you attack it with patience and confidence!

In addition, if promise to help anyone who is willing to learn with this topic! Comment, email, call, or coffee!

---------------------------------------------------------------------------

**But first, thank you to science.jrank.org!!! (the website from which I obtained my knowledge) <a href="http://science.jrank.org/pages/51901/prior-probability.html">prior probability</a>

---------------------------------------------------------------------------

Here are two kinds of probabilities: A prior probability and a posterior probability.

1. Prior probability- “The probability attached to an event before certain data are obtained.”
2. Posterior probability- “the probability attached to it after the data are obtained.”

Because the information on the resurrection of Jesus has already been obtained, we are looking at a posterior probability.

To find a posterior probability, we use Baye’s Theorem:

Don’t get intimidated by this big equation! There is a simple way to break it down.

Pr(Ai|B) is the thing we are trying to find. It is the probability of an event, (Ai), happening with respect to other events B. A1 is one event, A2 is another event, A3 is another event, and we can just keep on adding as many events as we want. The total number of events we have in our probability is equal to K.

The best way to explain this will be to use an example. We will make it simple- only two events- A1 and A2.

• Let’s make A1 the tossing of a double sided coin- both sides are heads. We will call this coin “Coin X. ” The probability of getting ‘heads’ when tossing Coin X is 100%. Event probability: 100%.
• Let’s make A2 the tossing of a normal coin with heads on one side and tails on the other. We will call this coin “Coin Y.” The probability of getting ‘heads’ when tossing Coin Y is 50%. Event probability: 50%

Now, we are going to put the two coins into a bag, blindfold Larry, and ask him to pick one of the coins randomly from the bag. The probability that Larry will pick Coin X is 50%, and the probability that he will pick Coin Y is 50%.

Keep in mind that picking Coin X corresponds to the event “A1.” Picking Coin Y corresponds to “A2.”

The way to say that “the probability of Larry picking Coin 1 is 50%” is: Pr(A1)=1/2

The way to say that “the probability of Larry picking Coin 2 is 50%” is: Pr(A2)=1/2

Now, let’s make B the ‘event’ of obtaining heads after flipping the coin that Larry randomly selects.

• If Larry picks Coin 1, the probability of obtaining heads is 100%, or Pr(B|A1)=1
• If Larry picks Coin 2, the probability of obtaining heads is 50%, or Pr(B|A2)=1/2

We are reading to see the whole mathematical formula for our example.

What this mathematical thingy is saying is this: “given that ‘heads’ was the outcome, the probability that it was the double-headed coin that was flipped is 2/3.” (science.jrank.org)

----------------------------------------------------------------------------------------------------

Know what I think it is time for? ANOTHER EXAMPLE!!!

Or rather, same example, but let’s do it where we are finding Pr(A2|B) instead of Pr(A1|B).

Summary of Heads Example:

Pr(A1) = 1/2

Pr(A2) = 1/2

Pr(B|A1) = 1

Pr(B|A2) = 1/2

So, given that ‘heads’ was obtained, what is the probability that it was Coin 2 that was tossed?

Here is the mathymajigger:

Pr(A2|B)=               Pr(B|A2) Pr(A2)                

                  Pr(B|A2) Pr(A2) + Pr(B|A1) Pr(A1)

Now we plug in our numbers:

Pr(A2|B)=              ½ x ½           
                      (½  x ½) + (1 x ½)

And after we do some fun math we come out with Pr(A2|B)= 1/3

This means: Given that ‘heads’ was obtained, the probability that it was Coin 2 that was tossed is 1/3.

From our first two examples, we now know that if the event that occurs is obtaining heads (or B=’the event of obtaining heads), that the probability that it was Coin 1 that was flipped is 2/3, and the probability that it was Coin 2 that was flipped is 1/3.
-----------------------------------------------------------------------------------

Ok time for a break! NOT. TIME FOR ANOTHER EXAMPLE!!! MUAHAHAHAHAAHAAAA!

It seems like I woke up this morning and thought “I wonder what blog post I could write to scare off the most people, give the highest number of people headaches, and discourage basically everyone to never read anything Josh writes again? Oh! I know! I’ll do an excruciatingly long post on PROBABILITY CALCULUS!!! And then I’ll give them… As many examples as they will need to actually understand what I’m saying...”

Anyway,iIf you are like me, you NEED this many examples to understand these principles. In addition, IF YOU WANT TO KNOW WHETHER OR NOT IT IS PROBABLE THAT JESUS ROSE FROM THE DEAD, YOU NEED TO UNDERSTAND BAYE’S THEOREM. And I’m going to make it as easy as possible for you! I'm doing all the leg work!
----------------------------------------------------------------------------------

Ok, same example as before, but we are going to make C the event of obtaining ‘tails’ instead of using B.

So, given that ‘tails’ was obtained, what is the probability that it was Coin 1 that was tossed?

• Let’s make A1 the tossing of a double sided coin- both sides are heads. The probability of getting ‘tails’ when tossing Coin X is 0%. Event probability: 0%.
• Let’s make A2 the tossing of a normal coin with heads on one side and tails on the other. The probability of getting ‘tails' when tossing Coin Y is 50%. Event probability: 50%

----------------------------------

• The probability of Larry picking Coin 1 is 50%: Pr(A1)=1/2
• The probability of Larry picking Coin 2 is 50%: Pr(A2)=1/2
• If Larry picks Coin 1, the probability of obtaining tails is 0%, or Pr(C|A1)=0
• If Larry picks Coin 2, the probability of obtaining tails is 50%, or Pr(C|A2)=1/2

Summary of Tails Example:

Pr(A1) = 1/2

Pr(A2) = 1/2

Pr(B|A1) = 0

Pr(B|A2) = 1/2

Our mathematical thingy:
Pr(A1|C)=              Pr(C|A1) Pr(A1)                 
                  Pr(C|A1) Pr(A1) + Pr(C|A2) Pr(A2)

Now we plug in the numbers:
Pr(A1|C)=            0 x ½              
                   (0 x ½) + (½ x ½)

This means: Given that ‘tails’ was obtained, the probability that it was Coin 1 that was tossed is 0.

*This example is especially useful because you can use your intuition to find the answer without using math- ‘tails’ cannot be obtained from flipped a coin with heads on both sides. By relating your intuition to the math, you can increase your understanding of probabilities!
-----------------------------------------------------------------------------

WHO’S READY FOR ONE MORE EXAMPLE? Well, I’ve only been studying, writing, and trying to make this crazy math stuff understandable for the past 6 hours of my Saturday morning. Might as well be thorough.

This last example should be ridiculously easy if you read the first three.

Same example, but let’s see what the probability of Coin 2 being tossed is if the result is ‘tails.'

Summary of Tails Example:

Pr(A1) = 1/2

Pr(A2) = 1/2

Pr(B|A1) = 0

Pr(B|A2) = 1/2

And the summary in english:

• The probability of Larry picking Coin 1 is 50%: Pr(A1)=1/2
• The probability of Larry picking Coin 2 is 50%: Pr(A2)=1/2
• If Larry picks Coin 1, the probability of obtaining tails is 0%, or Pr(C|A1)=0
• If Larry picks Coin 2, the probability of obtaining tails is 50%, or Pr(C|A2)=1/2

Pr(A2|C)=              Pr(C|A2) Pr(A2)                 
                  Pr(C|A2) Pr(A2) + Pr(C|A1) Pr(A1)

Pr(A2|C)=             ½ x ½            
                     (½ x ½) + (0 x ½)

This means: Given that ‘tails’ was obtained, the probability that it was Coin 1 that was tossed is 1 (or 100%).

If you managed to make it this far in this post, the next time I see you, I WILL give you a hug. If you don’t want to be hugged, just tell me that you didn’t read the last word or two. Otherwise I WILL hug you.

So, what does this have to do with the resurrection of Jesus? Check out my next post on Craig’s rebuttal of Ehrman’s argument against the resurrection of Jesus. Now that you are equipped with ‘the sword of probability calculus,’ you will be able to understand what the heck William Lane Craig is actually talking about!

For more analysis of probability, and a greater focus on the implications for the Craig-Ehrman debate, check out this post by neo the philosopher.

Can a Miracle Ever Be the Best Explanation?

Can a miracle ever be the best explanation for a historical event?

Maybe.

I currently do not know of a single miracle that has been or even can be historically verified, but the resurrection of Jesus is a possibility.

In my latest post on history, The Failure of the Christological Argument? (http://honestsearchfortruth.blogspot.com/2011/08/failure-of-christological-argument.html) I outlined a potent argument from Dr. Ehrman against the Christological Argument for the existence of God. Ehrman's contention is that a miracle cannot be the best explanation of historical evidence because miracles are less likely than any of the natural explanations. Historians can only decide 'what most likely occurred in the past,' and even an unlikely or absurd natural explanation is more likely than a miraculous explanation. As such, historians are entirely unable to say that God miraculously raised Jesus from the dead, even if the natural explanations for the evidence are pathetic (read my summary or watch the debate on youtube for a comprehensive understanding of the argument).

If we were considering a serious dating/courting relationship with the Historical/Christological arguments at this point, would Dr. Ehrman's argument be considered a "deal breaker?" (Perhaps the Historical Argument doesn't want to have kids? Or wants to have 17 of them...) I think that a "deal breaker" is extremely difficult to find when it comes to making conclusions on deep topics (and what could be more in depth than the Historical Argument?). A full body of evidence must be examined before conclusions can be drawn.

At this point, if I had to bet my life on the issue right now, I would say "no, the historical evidence does not affirm the resurrection of Jesus." However, I am by no means satisfied to end the discussion on here. I don't have a firm grasp on early church history, I have only a basic understanding of the Jewish, Roman, and Pagan cultures surrounding the events, I am only on chapter 3 of my Greek book, I have not hyper analyzed every pro and anti argument available, and I have not yet formulated original arguments on the topic.

What we all need to make a decision on this issue (and every issue) is depth. Until all of the relevant evidence has been gathered and analyzed, no conclusion should be drawn. This principle is especially relevant for anyone who reads one book on the topic, or who reads only books from a single perspective, or who hears a few sermons or takes a single class on the historicity of the Gospels/Bible/resurrection. To decide that one has reached the truth on this issue without pouring over both sides of the argument would be utter folly. As such, I shall continue to read, read, read, read... and learn Greek.

Time to Learn Greek! Diphthongs and the 5 Cases!

I know that everyone is just itching to run to the nearest book store, shell out some dough for a Greek book, and devote hours of their time to learning an ancient language!

On top of all that, I bet you can't wait to hear about the topic of this post- diphthongs and the 5 cases of Greek!

I'm sure all of you have memorized the Greek alphabet already, but if you need a refresher, check this out:

And now on to diphthongs!

And finally, the 5 cases:

1. Nominative- case of the subject (or more precisely, the subject of the finite verb)
2. Genitive- case of possession, origin, and separation
3. Accusative- case of direct object
4. Dative- case of indirect object and of locative (locative means 'location,' so a spatial relationship) and instrumental relationships (it can help to think of instrumental as 'to use like a tool')
5. Vocative- case of direct address

I hope you have enjoyed!!!

Ευχαριστώ!